Prodigi
Dance, Phosphor Fine Art Print
Dance, Phosphor Fine Art Print
Couldn't load pickup availability
A minimal surface is a mathematical abstraction of a soap film.
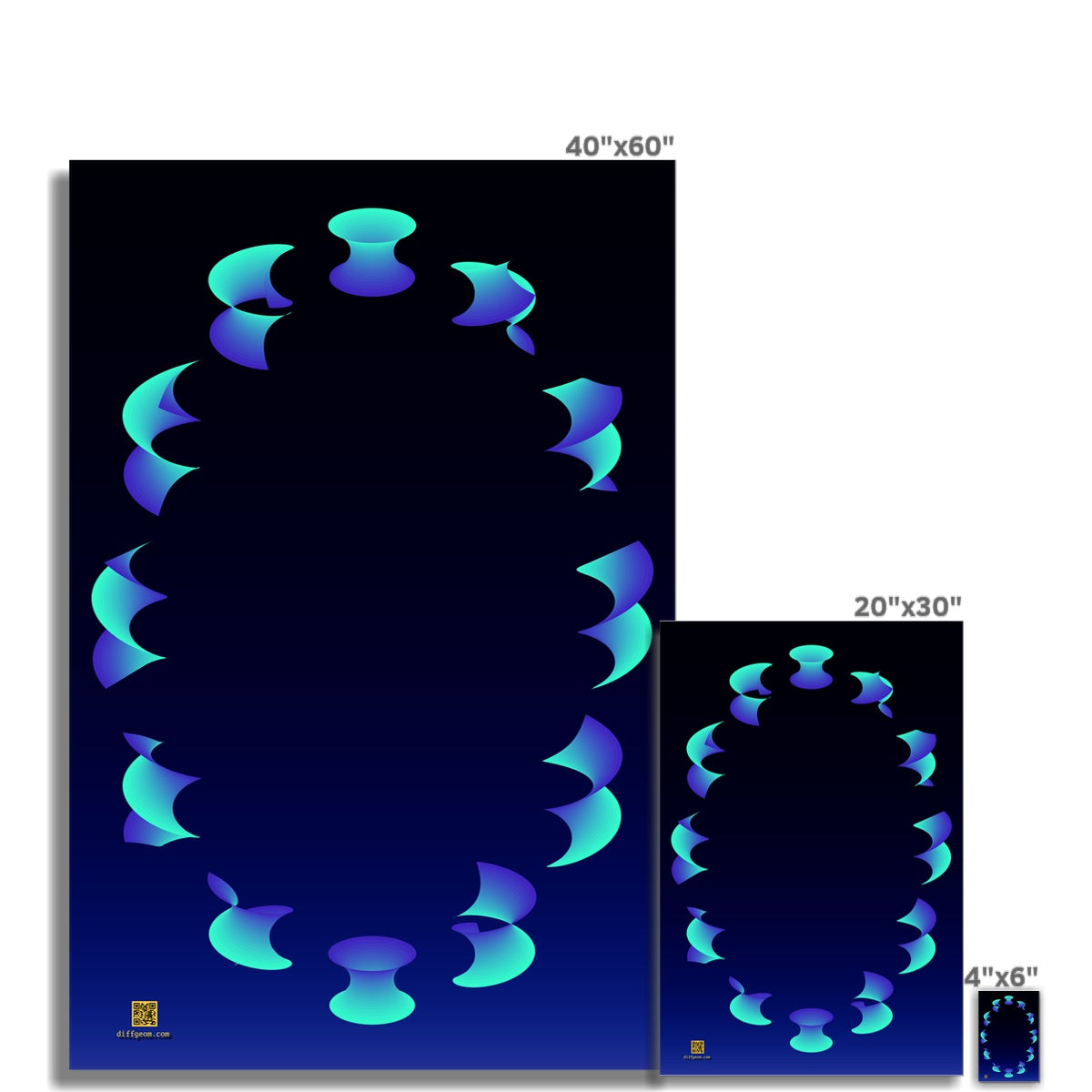
The catenoid is the surface of rotation swept by revolving the hyperbolic cosine graph \(x = \cosh z\) about the \(z\)-axis. The catenoid is minimal. Inversely, it has been known since the 18th century that (up to scaling) the catenoid is the only "complete" non-planar minimal surface of rotation.
The helicoid is the surface swept by a line \(L\) orthogonal to the \(z\)-axis in three-space if we rotate \(L\) at unit angular speed about the \(z\)-axis while simultaneously translating \(L\) at unit speed along the \(z\)-axis. Mathematicians call the helicoid a "ruled" surface because it is a union of lines. The helicoid is minimal. Inversely, it has been known since the 18th century that (up to scaling) the helicoid is the only "complete" non-planar minimal ruled surface.
Remarkably, one full turn of the helicoid can be wrapped around the catenoid without stretching or folding; there is a "local isometry" from the helicoid onto the catenoid.
More is true: The catenoid and helicoid are the real and imaginary parts of an abstract circular family of surfaces in complex three-space. When this family is projected to real three-space by rotating through the complex circle parameter and taking the real part, we get the family of minimal surfaces shown.
Printed on museum-quality fine art print paper (200 gsm) with a textured, matte finish.
Share