Kuen's Surface, Aqua Twilight Wall Art Poster

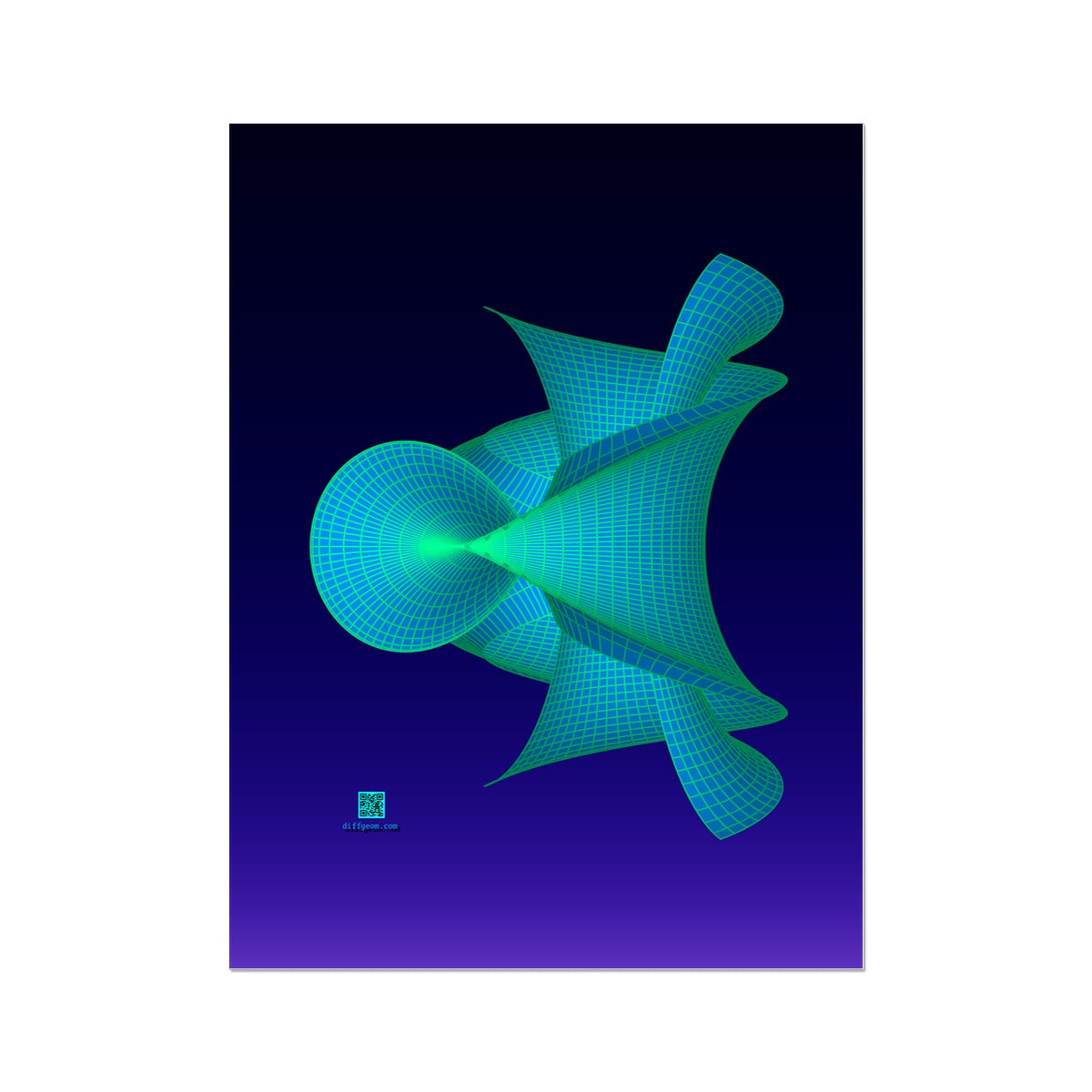
On a surface in Euclidean three-space, straightest paths, those of locally-shortest length, are called "geodesics." Kuen's surface has "Gaussian curvature" equal to \(-1\) (away from curves where the surface pinches to a sharp edge). Concretely, for every geodesic triangle on the surface, the sum of the interior angles (in radians) is equal to \(\pi\) minus the area of the triangle. On a surface in Euclidean three-space, "straightest" paths, those of locally-shortest length, are called "geodesics." Geodesic triangles on a surface generally do not have total interior angle equal to a straight angle: The "angular defect," the sum of the interior angles (in radians) minus \(\pi\), is generally non-zero. For triangles with short sides the angular defect is nearly proportional to the area. If we fix a point \(p\) and consider geodesic triangles containing \(p\), the limiting constant of proportionality as the sides approach \(0\) is the "Gaussian curvature" at \(p\). Kuen's surface has Gaussian curvature equal to \(-1\) at each point (except for curves where the surface pinches to a sharp edge), and is consequently a model for small pieces of the hyperbolic plane.

Printed on high-quality 170 gsm poster paper with a satin finish.