Nowhere Monotone Function, Dark Wall Art Poster

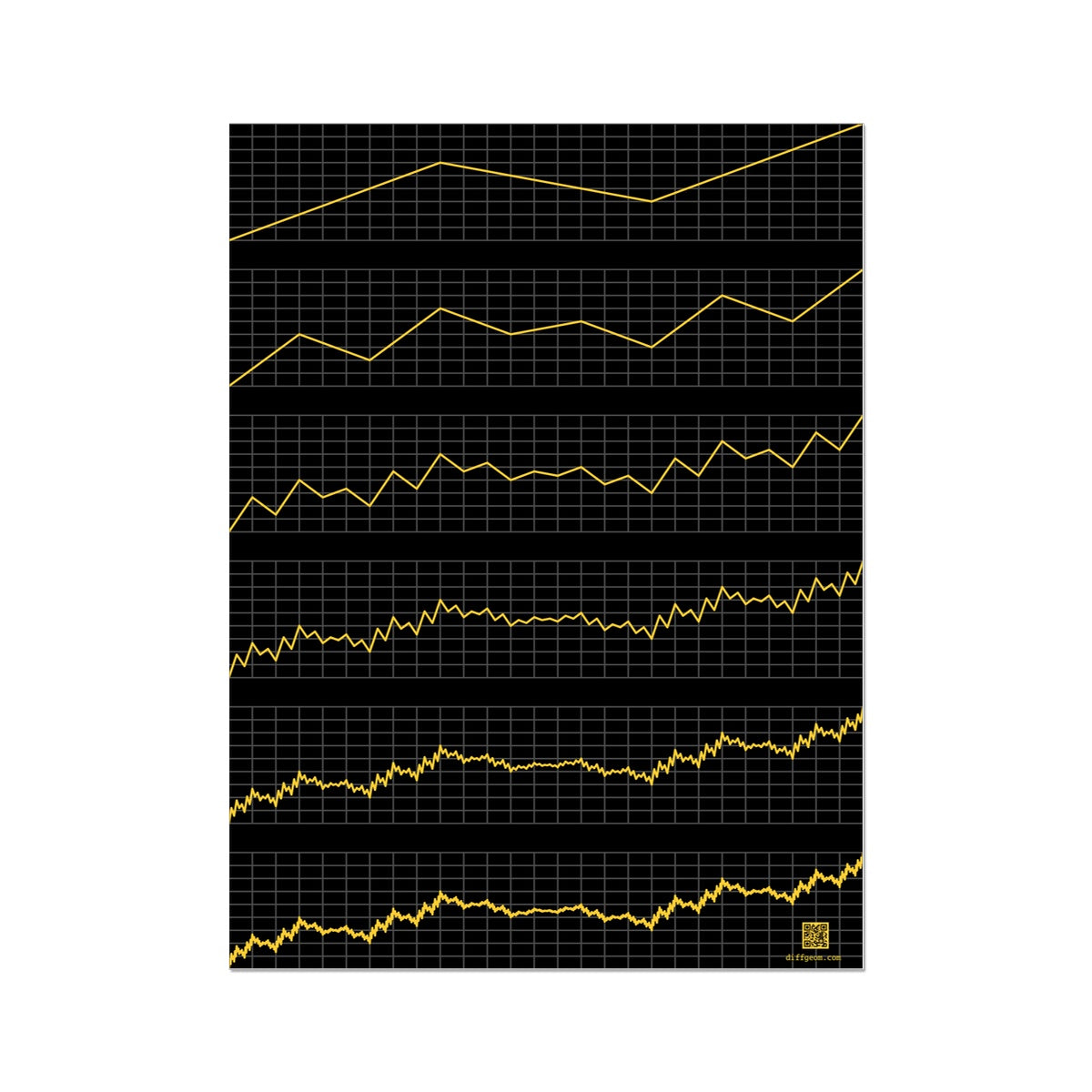
Calculus instructors often use the phrase ``continuous function'' (loosely, one whose graph has no jumps) while drawing a differentiable function (one whose graph has a tangent line at each point). It can therefore come as a conceptual shock to learn in real analysis that a continuous function may be differentiable nowhere, and in fact that a ``randomly chosen'' continuous function is nowhere-differentiable.

A sequence of piecewise-linear (connect-the-dots) functions can converge to a nowhere-monotone, hence nowhere-differentiable, function. In the image, a sequence of functions is defined recursively by replacing each line segment with a zig-zag having the same endpoints. Such a sequence converges ``uniformly'' to a continuous limit.

Printed on high-quality 170 gsm poster paper with a satin finish.